ত্রিকোণমিতিক অভেদসমূহের তালিকা

ত্রিকনমিতিতে, ত্রিকোণমিতিক সুত্রসমূহ হল এমন সমীকরণ যা ত্রিকোণমিতিক ফাংশনগুলিকে জড়িত করে এবং যেগুলির জন্য সমতার উভয় দিককে সংজ্ঞায়িত করা হয় সেই চলকগুলির প্রতিটি মানের জন্য সত্য ৷ জ্যামিতিকভাবে, এগুলি এক বা একাধিক কোণের নির্দিষ্ট ফাংশন জড়িত অভেদ। এগুলি ত্রিভুজের অভেদ থেকে আলাদা, যেগুলি সম্ভাব্য কোণ জড়িত কিন্তু পার্শ্ব দৈর্ঘ্য বা ত্রিভুজের অন্যান্য দৈর্ঘ্যও জড়িত।

যখনই ত্রিকোণমিতিক ফাংশন জড়িত রাশিকে সরলীকরণের প্রয়োজন হয় তখন এই সূত্রগুলি কার্যকর। একটি গুরুত্বপূর্ণ প্রয়োগ হল অ-ত্রিকোণমিতিক ফাংশনগুলির যোগজীকরণ, একটি সাধারণ কৌশলের মধ্যে প্রথমে ত্রিকোণমিতিক প্রতিস্থাপন ব্যবহার করে এবং তারপর ত্রিকোণমিতিক সূত্রের সাথে প্রাপ্ত অবিচ্ছেদ্যকে সরল করা হয়।

পিথাগোরীয় অভেদসমূহ

মূল নিবন্ধ: পিথাগোরিয়ান ত্রিকোণমিতিক অভেদসমূহ

একক বৃত্তে ত্রিকোণমিতিক ফাংশন এবং তাদের গুণক বিপরীত । সমকোণী ত্রিভুজের সবগুলোই একই রকম, অর্থাৎ তাদের সংশ্লিষ্ট পাশের মধ্যে অনুপাত একই। সাইন, কোসাইন এবং টেনজেন্ট-এর জন্য একক-দৈর্ঘ্য ব্যাসার্ধ ত্রিভুজের কর্ণ গঠন করে যা তাদের সংজ্ঞায়িত করে। গুণক বিপরীত পরিচয়গুলি ত্রিভুজের বাহুর অনুপাত হিসাবে উদ্ভূত হয় যেখানে এই একক রেখাটি আর কর্ণ নয়। নীল ত্রিভুজটি এই পরিচয়টি চিত্রিত করে $1+\cot ^{2}\theta =\csc ^{2}\theta$, এবং লাল ত্রিভুজ দেখায় যে $\tan ^{2}\theta +1=\sec ^{2}\theta$.

সাইন এবং কোসাইন-এর মধ্যে মৌলিক সম্পর্ক নিম্ন পিথাগোরীয় অভেদ দ্বারা দেওয়া হয়েছে:

$$\sin ^{2}\theta +\cos ^{2}\theta =1,$$

যেখানে $\sin ^{2}\theta$ মানে $(\sin \theta )^{2}$ এবং $\cos ^{2}\theta$ মানে $(\cos \theta )^{2}$ ।

এটি পিথাগোরিয়ান উপপাদ্যের একটি সংস্করণ হিসাবে দেখা যেতে পারে এবং নিম্ন সমীকরণ থেকে অনুসরণ করে $x^{2}+y^{2}=1$ একক বৃত্তের জন্য । এই সমীকরণটি সাইন বা কোসাইনের জন্য সমাধান করা যেতে পারে:

$${\begin{aligned}\sin \theta &=\pm {\sqrt {1-\cos ^{2}\theta }},\\\cos \theta &=\pm {\sqrt {1-\sin ^{2}\theta }}.\end{aligned}}$$

যেখানে চিহ্নটি $\theta$ এর বৃত্তের এক-চতুর্থাংশ এর উপর নির্ভর করে।

এই অভেদকে $\sin ^{2}\theta$, $\cos ^{2}\theta$, বা উভয় দ্বারা ভাগ করলে নিম্নলিখিত অভেদগুলো পাওয়া যায়: $${\begin{aligned}&1+\cot ^{2}\theta =\csc ^{2}\theta \\&1+\tan ^{2}\theta =\sec ^{2}\theta \\&\sec ^{2}\theta +\csc ^{2}\theta =\sec ^{2}\theta \csc ^{2}\theta \end{aligned}}$$

এই অভেদগুলি ব্যবহার করে যেকোনো ত্রিকোণমিতিক ফাংশনকে অন্য যেকোনো পরিপ্রেক্ষিতে প্রকাশ করা সম্ভব ।

অন্য পাঁচটির প্রতিটির পরিপ্রেক্ষিতে প্রতিটি ত্রিকোণমিতিক ফাংশন.

পরিপ্রেক্ষিতে	$\sin \theta$	$\csc \theta$	$\cos \theta$	$\sec \theta$	$\tan \theta$	$\cot \theta$
$\sin \theta =$	$\sin \theta$	${\frac {1}{\csc \theta }}$	$\pm {\sqrt {1-\cos ^{2}\theta }}$	$\pm {\frac {\sqrt {\sec ^{2}\theta -1}}{\sec \theta }}$	$\pm {\frac {\tan \theta }{\sqrt {1+\tan ^{2}\theta }}}$	$\pm {\frac {1}{\sqrt {1+\cot ^{2}\theta }}}$
$\csc \theta =$	${\frac {1}{\sin \theta }}$	$\csc \theta$	$\pm {\frac {1}{\sqrt {1-\cos ^{2}\theta }}}$	$\pm {\frac {\sec \theta }{\sqrt {\sec ^{2}\theta -1}}}$	$\pm {\frac {\sqrt {1+\tan ^{2}\theta }}{\tan \theta }}$	$\pm {\sqrt {1+\cot ^{2}\theta }}$
$\cos \theta =$	$\pm {\sqrt {1-\sin ^{2}\theta }}$	$\pm {\frac {\sqrt {\csc ^{2}\theta -1}}{\csc \theta }}$	$\cos \theta$	${\frac {1}{\sec \theta }}$	$\pm {\frac {1}{\sqrt {1+\tan ^{2}\theta }}}$	$\pm {\frac {\cot \theta }{\sqrt {1+\cot ^{2}\theta }}}$
$\sec \theta =$	$\pm {\frac {1}{\sqrt {1-\sin ^{2}\theta }}}$	$\pm {\frac {\csc \theta }{\sqrt {\csc ^{2}\theta -1}}}$	${\frac {1}{\cos \theta }}$	$\sec \theta$	$\pm {\sqrt {1+\tan ^{2}\theta }}$	$\pm {\frac {\sqrt {1+\cot ^{2}\theta }}{\cot \theta }}$
$\tan \theta =$	$\pm {\frac {\sin \theta }{\sqrt {1-\sin ^{2}\theta }}}$	$\pm {\frac {1}{\sqrt {\csc ^{2}\theta -1}}}$	$\pm {\frac {\sqrt {1-\cos ^{2}\theta }}{\cos \theta }}$	$\pm {\sqrt {\sec ^{2}\theta -1}}$	$\tan \theta$	${\frac {1}{\cot \theta }}$
$\cot \theta =$	$\pm {\frac {\sqrt {1-\sin ^{2}\theta }}{\sin \theta }}$	$\pm {\sqrt {\csc ^{2}\theta -1}}$	$\pm {\frac {\cos \theta }{\sqrt {1-\cos ^{2}\theta }}}$	$\pm {\frac {1}{\sqrt {\sec ^{2}\theta -1}}}$	${\frac {1}{\tan \theta }}$	$\cot \theta$

প্রতিফলন, পরিবর্তন, এবং পর্যায়ক্রম

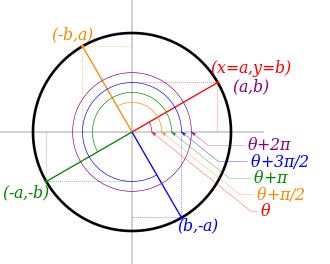
একক বৃত্ত পরীক্ষা করে, কেউ ত্রিকোণমিতিক ফাংশনগুলির নিম্নলিখিত বৈশিষ্ট্যগুলি স্থাপন করতে পারে।

প্রতিফলন

${\frac {\pi }{4}}$ এর বৃদ্ধিতে প্রতিফলন কোণ $\alpha$ স্থানান্তরিত করার সময় স্থানাঙ্কের রূপান্তর (a, b)।

যখন একটি ইউক্লিডীয় ভেক্টরের দিক একটি কোণ $\theta ,$ দ্বারা উপস্থাপিত হয় তখন এটি মুক্ত ভেক্টর (উৎপত্তি থেকে শুরু করে) এবং ধনাত্মক $x$-একক ভেক্টর দ্বারা নির্ধারিত কোণ। একই ধারণা ইউক্লিডীয় স্থানের রেখার ক্ষেত্রেও প্রয়োগ করা যেতে পারে, যেখানে কোণটি উৎপত্তি এবং ধনাত্মক x-অক্ষের মাধ্যমে প্রদত্ত রেখার সমান্তরাল দ্বারা নির্ধারিত হয় । যদি $\theta$ দিকনির্দেশ সহ একটি রেখা (ভেক্টর) $\alpha ,$ দিক সহ একটি রেখা সম্পর্কে প্রতিফলিত হয় তবে দিক কোণ $\theta ^{\prime }$ এই প্রতিফলিত লাইনের (ভেক্টর) মান হচ্ছে $$\theta ^{\prime }=2\alpha -\theta .$$ ।

এই কোণগুলির ত্রিকোণমিতিক ফাংশনের মান $\theta ,\;\theta ^{\prime }$ নির্দিষ্ট কোণগুলির জন্য $\alpha$ সরল পরিচয়কে সন্তুষ্ট করে: হয় তারা সমান, অথবা বিপরীত চিহ্ন আছে, বা পরিপূরক ত্রিকোণমিতিক ফাংশন নিয়োগ. এগুলি হ্রাস সূত্র নামেও পরিচিত৷ ।

$\theta$ প্রতিফলিত $\alpha =0$ odd/even identities	$\theta$ প্রতিফলিত $\alpha ={\frac {\pi }{4}}$	$\theta$ প্রতিফলিত $\alpha ={\frac {\pi }{2}}$	$\theta$ প্রতিফলিত $\alpha ={\frac {3\pi }{4}}$	$\theta$ প্রতিফলিত $\alpha =\pi$ compare to $\alpha =0$
$\sin(-\theta )=-\sin \theta$	$\sin \left({\tfrac {\pi }{2}}-\theta \right)=\cos \theta$	$\sin(\pi -\theta )=+\sin \theta$	$\sin \left({\tfrac {3\pi }{2}}-\theta \right)=-\cos \theta$	$\sin(2\pi -\theta )=-\sin(\theta )=\sin(-\theta )$
$\cos(-\theta )=+\cos \theta$	$\cos \left({\tfrac {\pi }{2}}-\theta \right)=\sin \theta$	$\cos(\pi -\theta )=-\cos \theta$	$\cos \left({\tfrac {3\pi }{2}}-\theta \right)=-\sin \theta$	$\cos(2\pi -\theta )=+\cos(\theta )=\cos(-\theta )$
$\tan(-\theta )=-\tan \theta$	$\tan \left({\tfrac {\pi }{2}}-\theta \right)=\cot \theta$	$\tan(\pi -\theta )=-\tan \theta$	$\tan \left({\tfrac {3\pi }{2}}-\theta \right)=+\cot \theta$	$\tan(2\pi -\theta )=-\tan(\theta )=\tan(-\theta )$
$\csc(-\theta )=-\csc \theta$	$\csc \left({\tfrac {\pi }{2}}-\theta \right)=\sec \theta$	$\csc(\pi -\theta )=+\csc \theta$	$\csc \left({\tfrac {3\pi }{2}}-\theta \right)=-\sec \theta$	$\csc(2\pi -\theta )=-\csc(\theta )=\csc(-\theta )$
$\sec(-\theta )=+\sec \theta$	$\sec \left({\tfrac {\pi }{2}}-\theta \right)=\csc \theta$	$\sec(\pi -\theta )=-\sec \theta$	$\sec \left({\tfrac {3\pi }{2}}-\theta \right)=-\csc \theta$	$\sec(2\pi -\theta )=+\sec(\theta )=\sec(-\theta )$
$\cot(-\theta )=-\cot \theta$	$\cot \left({\tfrac {\pi }{2}}-\theta \right)=\tan \theta$	$\cot(\pi -\theta )=-\cot \theta$	$\cot \left({\tfrac {3\pi }{2}}-\theta \right)=+\tan \theta$	$\cot(2\pi -\theta )=-\cot(\theta )=\cot(-\theta )$

স্থানান্তর এবং পর্যায়ক্রম

${\frac {\pi }{2}}$-এর বৃদ্ধিতে কোণ $\theta$ স্থানান্তর করার সময় স্থানাঙ্কের রূপান্তর (a, b)।

এক চতুর্থাংশ পর্যায় দ্বারা স্থানান্তর	অর্ধেক পর্যায় দ্বারা স্থানান্তর	সম্পূর্ণ পর্যায় দ্বারা স্থানান্তর করুন	পর্যায়
$\sin(\theta \pm {\tfrac {\pi }{2}})=\pm \cos \theta$	$\sin(\theta +\pi )=-\sin \theta$	$\sin(\theta +k\cdot 2\pi )=+\sin \theta$	$2\pi$
$\cos(\theta \pm {\tfrac {\pi }{2}})=\mp \sin \theta$	$\cos(\theta +\pi )=-\cos \theta$	$\cos(\theta +k\cdot 2\pi )=+\cos \theta$	$2\pi$
$\csc(\theta \pm {\tfrac {\pi }{2}})=\pm \sec \theta$	$\csc(\theta +\pi )=-\csc \theta$	$\csc(\theta +k\cdot 2\pi )=+\csc \theta$	$2\pi$
$\sec(\theta \pm {\tfrac {\pi }{2}})=\mp \csc \theta$	$\sec(\theta +\pi )=-\sec \theta$	$\sec(\theta +k\cdot 2\pi )=+\sec \theta$	$2\pi$
$\tan(\theta \pm {\tfrac {\pi }{4}})={\tfrac {\tan \theta \pm 1}{1\mp \tan \theta }}$	$\tan(\theta +{\tfrac {\pi }{2}})=-\cot \theta$	$\tan(\theta +k\cdot \pi )=+\tan \theta$	$\pi$
$\cot(\theta \pm {\tfrac {\pi }{4}})={\tfrac {\cot \theta \mp 1}{1\pm \cot \theta }}$	$\cot(\theta +{\tfrac {\pi }{2}})=-\tan \theta$	$\cot(\theta +k\cdot \pi )=+\cot \theta$	$\pi$

চিহ্ন

ত্রিকোণমিতিক ফাংশনের চিহ্ন কোণের চতুর্ভুজের উপর নির্ভর করে । যদি ${-\pi }<\theta \leq \pi$ এবং sgn হয় চিহ্ন ফাংশন, তাহলে:

$${\begin{aligned}\operatorname {sgn}(\sin \theta )=\operatorname {sgn}(\csc \theta )&={\begin{cases}+1&{\text{if}}\ \ 0<\theta <\pi \\-1&{\text{if}}\ \ {-\pi }<\theta <0\\0&{\text{if}}\ \ \theta \in \{0,\pi \}\end{cases}}\\[5mu]\operatorname {sgn}(\cos \theta )=\operatorname {sgn}(\sec \theta )&={\begin{cases}+1&{\text{if}}\ \ {-{\tfrac {1}{2}}\pi }<\theta <{\tfrac {1}{2}}\pi \\-1&{\text{if}}\ \ {-\pi }<\theta <-{\tfrac {1}{2}}\pi \ \ {\text{or}}\ \ {\tfrac {1}{2}}\pi <\theta <\pi \\0&{\text{if}}\ \ \theta \in {\bigl \{}{-{\tfrac {1}{2}}\pi },{\tfrac {1}{2}}\pi {\bigr \}}\end{cases}}\\[5mu]\operatorname {sgn}(\tan \theta )=\operatorname {sgn}(\cot \theta )&={\begin{cases}+1&{\text{if}}\ \ {-\pi }<\theta <-{\tfrac {1}{2}}\pi \ \ {\text{or}}\ \ 0<\theta <{\tfrac {1}{2}}\pi \\-1&{\text{if}}\ \ {-{\tfrac {1}{2}}\pi }<\theta <0\ \ {\text{or}}\ \ {\tfrac {1}{2}}\pi <\theta <\pi \\0&{\text{if}}\ \ \theta \in {\bigl \{}{-{\tfrac {1}{2}}\pi },0,{\tfrac {1}{2}}\pi ,\pi {\bigr \}}\end{cases}}\end{aligned}}$$

ত্রিকোণমিতিক ফাংশনগুলি সাধারণ সময়ের সাথে পর্যায়ক্রমিক হয় $2\pi ,$ তাই ব্যবধানের বাইরে θ এর মানের জন্য $({-\pi },\pi ],$ > তারা পুনরাবৃত্তির মান নেয় (উপরে § Shifts এবং পর্যায়ক্রম দেখুন)।

কোণ যোগফল এবং পার্থক্য পরিচয়

আরও দেখুন: ত্রিকোণমিতিক অভেদসমূহের প্রমাণ § কোণ যোগফল অভেদসমূহ, ও ছোট-কোণ আনুমানিক § কোণ যোগফল এবং পার্থক্য

তীব্র কোণের সাইন এবং কোসাইনের জন্য কোণ যোগ সূত্রের চিত্রণ। জোর দেওয়া অংশটি একক দৈর্ঘ্যের।

$\sin(\alpha -\beta )$ এবং $\cos(\alpha -\beta )$-এর জন্য কোণ পার্থক্য পরিচয় দেখানো চিত্র।

এগুলি কোণ যোগ এবং বিয়োগ উপপাদ্য (বা সূত্র) নামেও পরিচিত। $${\begin{aligned}\sin(\alpha +\beta )&=\sin \alpha \cos \beta +\cos \alpha \sin \beta \\\sin(\alpha -\beta )&=\sin \alpha \cos \beta -\cos \alpha \sin \beta \\\cos(\alpha +\beta )&=\cos \alpha \cos \beta -\sin \alpha \sin \beta \\\cos(\alpha -\beta )&=\cos \alpha \cos \beta +\sin \alpha \sin \beta \end{aligned}}$$

$\sin(\alpha -\beta )$ এবং $\cos(\alpha -\beta )$-এর কোণ পার্থক্য অভেদগুলি $\beta$ এর জন্য >-\beta</math> এবং $\sin(-\beta )=-\sin(\beta )$ এবং $\ cos(-\beta )=\cos(\beta )$. কোণ সমষ্টি অভেদের জন্য চিত্রের একটি সামান্য পরিবর্তিত সংস্করণ ব্যবহার করেও সেগুলি বের করা যেতে পারে, উভয়ই এখানে দেখানো হয়েছে।

এই অভেদগুলি নিম্নলিখিত সারণীর প্রথম দুটি সারিতে সংক্ষিপ্ত করা হয়েছে, এতে অন্যান্য ত্রিকোণমিতিক ফাংশনের যোগফল এবং পার্থক্যও অন্তর্ভুক্ত রয়েছে।

সাইন	$\sin(\alpha \pm \beta )$			$=$	$\sin \alpha \cos \beta \pm \cos \alpha \sin \beta$
কোসাইন	$\cos(\alpha \pm \beta )$			$=$	$\cos \alpha \cos \beta \mp \sin \alpha \sin \beta$
ট্যাঞ্জেন্ট	$\tan(\alpha \pm \beta )$			$=$	${\frac {\tan \alpha \pm \tan \beta }{1\mp \tan \alpha \tan \beta }}$
কোসেক্যান্ট	$\csc(\alpha \pm \beta )$			$=$	${\frac {\sec \alpha \sec \beta \csc \alpha \csc \beta }{\sec \alpha \csc \beta \pm \csc \alpha \sec \beta }}$
সেক্যান্ট	$\sec(\alpha \pm \beta )$			$=$	${\frac {\sec \alpha \sec \beta \csc \alpha \csc \beta }{\csc \alpha \csc \beta \mp \sec \alpha \sec \beta }}$
কোট্যাঞ্জেন্ট	$\cot(\alpha \pm \beta )$			$=$	${\frac {\cot \alpha \cot \beta \mp 1}{\cot \beta \pm \cot \alpha }}$
আর্ক সাইন	$\arcsin x\pm \arcsin y$			$=$	$\arcsin \left(x{\sqrt {1-y^{2}}}\pm y{\sqrt {1-x^{2}}}\right)$
আর্ক কোসাইন	$\arccos x\pm \arccos y$			$=$	$\arccos \left(xy\mp {\sqrt {\left(1-x^{2}\right)\left(1-y^{2}\right)}}\right)$
আর্ক ট্যাঞ্জেন্ট	$\arctan x\pm \arctan y$			$=$	$\arctan \left({\frac {x\pm y}{1\mp xy}}\right)$
আর্ক কোট্যাঞ্জেন্ট	$\operatorname {arccot} x\pm \operatorname {arccot} y$			$=$	$\operatorname {arccot} \left({\frac {xy\mp 1}{y\pm x}}\right)$

বহু-কোণ এবং অর্ধ-কোণ সূত্র

Tn হল nতম চেবিশেভ বহুপদী	$\cos(n\theta )=T_{n}(\cos \theta )$
ডি মোইভারের সূত্র, i হল কাল্পনিক একক	$\cos(n\theta )+i\sin(n\theta )=(\cos \theta +i\sin \theta )^{n}$

বহু-কোণ সূত্র

দ্বি-কোণ সূত্র

সাইনের জন্য দ্বি-কোণ সূত্রের ভিজ্যুয়াল প্রদর্শন। একটি সমদ্বিবাহু ত্রিভুজের ক্ষেত্রফল, +১/২ × বেস × উচ্চতা গণনা করা হয়, প্রথমে যখন খাড়া থাকে এবং তারপরে তার পাশে থাকে। সোজা হলে, এলাকা = $\sin \theta \cos \theta$ । যখন এর পাশে, এলাকা = ${\textstyle {\frac {1}{2}}\sin 2\theta }$। ত্রিভুজ ঘোরানো তার ক্ষেত্রফল পরিবর্তন করে না, তাই এই দুটি রাশি সমান। অতএব, $\sin 2\theta =2\sin \theta \cos \theta$ ।

দ্বিগুণ কোণের জন্য সূত্র।

• $\sin(2\theta )=2\sin \theta \cos \theta =(\sin \theta +\cos \theta )^{2}-1={\frac {2\tan \theta }{1+\tan ^{2}\theta }}$
• $\cos(2\theta )=\cos ^{2}\theta -\sin ^{2}\theta =2\cos ^{2}\theta -1=1-2\sin ^{2}\theta ={\frac {1-\tan ^{2}\theta }{1+\tan ^{2}\theta }}$
• $\tan(2\theta )={\frac {2\tan \theta }{1-\tan ^{2}\theta }}$
• $\cot(2\theta )={\frac {\cot ^{2}\theta -1}{2\cot \theta }}={\frac {1-\tan ^{2}\theta }{2\tan \theta }}$
• $\sec(2\theta )={\frac {\sec ^{2}\theta }{2-\sec ^{2}\theta }}={\frac {1+\tan ^{2}\theta }{1-\tan ^{2}\theta }}$
• $\csc(2\theta )={\frac {\sec \theta \csc \theta }{2}}={\frac {1+\tan ^{2}\theta }{2\tan \theta }}$

ত্রি-কোণ সূত্র

ট্রিপল অ্যাঙ্গেলের সূত্র।

• $\sin(3\theta )=3\sin \theta -4\sin ^{3}\theta =4\sin \theta \sin \left({\frac {\pi }{3}}-\theta \right)\sin \left({\frac {\pi }{3}}+\theta \right)$
• $\cos(3\theta )=4\cos ^{3}\theta -3\cos \theta =4\cos \theta \cos \left({\frac {\pi }{3}}-\theta \right)\cos \left({\frac {\pi }{3}}+\theta \right)$
• $\tan(3\theta )={\frac {3\tan \theta -\tan ^{3}\theta }{1-3\tan ^{2}\theta }}=\tan \theta \tan \left({\frac {\pi }{3}}-\theta \right)\tan \left({\frac {\pi }{3}}+\theta \right)$
• $\cot(3\theta )={\frac {3\cot \theta -\cot ^{3}\theta }{1-3\cot ^{2}\theta }}$
• $\sec(3\theta )={\frac {\sec ^{3}\theta }{4-3\sec ^{2}\theta }}$
• $\csc(3\theta )={\frac {\csc ^{3}\theta }{3\csc ^{2}\theta -4}}$

বহু-কোণ সূত্র

• ${\begin{aligned}\sin(n\theta )&=\sum _{k{\text{ odd}}}(-1)^{\frac {k-1}{2}}{n \choose k}\cos ^{n-k}\theta \sin ^{k}\theta =\sin \theta \sum _{i=0}^{(n+1)/2}\sum _{j=0}^{i}(-1)^{i-j}{n \choose 2i+1}{i \choose j}\cos ^{n-2(i-j)-1}\theta \\{}&=2^{(n-1)}\prod _{k=0}^{n-1}\sin(k\pi /n+\theta )\end{aligned}}$
• $\cos(n\theta )=\sum _{k{\text{ even}}}(-1)^{\frac {k}{2}}{n \choose k}\cos ^{n-k}\theta \sin ^{k}\theta =\sum _{i=0}^{n/2}\sum _{j=0}^{i}(-1)^{i-j}{n \choose 2i}{i \choose j}\cos ^{n-2(i-j)}\theta$
• $\cos((2n+1)\theta )=(-1)^{n}2^{2n}\prod _{k=0}^{2n}\cos(k\pi /(2n+1)-\theta )$
• $\cos(2n\theta )=(-1)^{n}2^{2n-1}\prod _{k=0}^{2n-1}\cos((1+2k)\pi /(4n)-\theta )$
• $\tan(n\theta )={\frac {\sum _{k{\text{ odd}}}(-1)^{\frac {k-1}{2}}{n \choose k}\tan ^{k}\theta }{\sum _{k{\text{ even}}}(-1)^{\frac {k}{2}}{n \choose k}\tan ^{k}\theta }}$