বিশৃঙ্খলা তত্ত্ব

গণিত ও পদার্থবিজ্ঞানে বিশৃঙ্খলা তত্ত্ব (ইংরেজি: chaos theory) কতগুলি অ-যোগাশ্রয়ী গতিশীল ব্যবস্থার আচরণ বর্ণনা করে। এই ব্যবস্থাগুলি কিছু শর্তের অধীনে এমন এক ধরনের গতিশীল আচরণ প্রদর্শন করে যেগুলি প্রাথমিক শর্তের উপর সংবেদনশীল। এই সংবেদনশীলতার কারণে বিশৃঙ্খল ব্যবস্থাগুলির আচরণ অনির্দিষ্ট বা দৈব (random) বলে মনে হয়[১][২], কারণ প্রাথমিক শর্তগুলিতে অবস্থিত ত্রুটিগুলিতে সূচকীয় বৃদ্ধি (exponential growth) ঘটে। এই ব্যবস্থাগুলি নিয়তিবাদী (deterministic) এই অর্থে যে তাদের ভবিষ্যৎ গতিময়তা প্রাথমিক শর্তগুলি দ্বারা সুসংজ্ঞায়িত। কিন্তু তারপরেও এগুলিতে বিশৃঙ্খলা ঘটে।
পরীক্ষাগারে বিভিন্ন ব্যবস্থায় বিশৃঙ্খল আচরণ পরিলক্ষিত হয়েছে। এদের মধ্যে আছে বৈদ্যুতিক বর্তনী, লেজার, রাসায়নিক বিক্রিয়া, প্রবাহী গতিবিদ্যা, এবং বিভিন্ন যান্ত্রিক ও চৌম্বক-যান্ত্রিক যন্ত্র। সৌরজগতের উপগ্রহগুলির গতি, বাস্তুব্যবস্থায় জনসংখ্যার বৃদ্ধি, নিউরনের সক্রিয়ন বিভব, আণবিক স্পন্দন, আবহাওয়া ও জলবায়ু [৩], ইত্যাদি প্রাকৃতিক ঘটনাবলিতেও গাণিতিক বিশৃঙ্খলা দেখতে পাওয়া যায়।
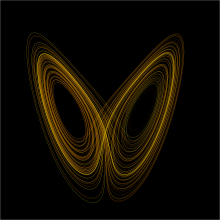
গাণিতিক বিশৃঙ্খলা প্রদর্শন করে এমন ব্যবস্থাগুলি নিয়তিবাদী (deterministic) বলে এগুলোর মধ্যে এক অর্থে শৃঙ্খলা আছে। অর্থাৎ এগুলি পুরোপুরি বিশৃঙ্খল নয়। এই ব্যবস্থাগুলির পরিসংখ্যানিক আচরণও সুসংজ্ঞায়িত। উদাহরণস্বরূপ, চিত্রের লোরেন্ৎস ব্যবস্থাটি গাণিতিকভাবে বিশৃঙ্খল, কিন্তু এর কাঠামো সুসংজ্ঞায়িত।
তথ্যসূত্র
[সম্পাদনা]- ↑ Kellert, Stephen H. (১৯৯৩)। In the Wake of Chaos: Unpredictable Order in Dynamical Systems। University of Chicago Press। পৃষ্ঠা 32। আইএসবিএন 978-0-226-42976-2।
- ↑ Boeing, G. (২০১৬)। "Visual Analysis of Nonlinear Dynamical Systems: Chaos, Fractals, Self-Similarity and the Limits of Prediction"। Systems। 4 (4): 37। ডিওআই:10.3390/systems4040037। সংগ্রহের তারিখ ২০১৬-১২-০২।
- ↑ Sneyers, R: "Climate Chaotic Instability: Statistical Determination and Theoretical Background", 8(5):517-532
বহিসংযোগ
[সম্পাদনা]- Hazewinkel, Michiel, সম্পাদক (২০০১), "Chaos", Encyclopedia of Mathematics, Springer Science+Business Media, আইএসবিএন 978-1-55608-010-4
- Nonlinear Dynamics Research Group with Animations in Flash
- The Chaos group at the University of Maryland
- The Chaos Hypertextbook. An introductory primer on chaos and fractals
- ChaosBook.org An advanced graduate textbook on chaos (no fractals)
- Society for Chaos Theory in Psychology & Life Sciences
- Nonlinear Dynamics Research Group at CSDC, Florence Italy
- Interactive live chaotic pendulum experiment, allows users to interact and sample data from a real working damped driven chaotic pendulum
- Nonlinear dynamics: how science comprehends chaos, talk presented by Sunny Auyang, 1998.
- Nonlinear Dynamics. Models of bifurcation and chaos by Elmer G. Wiens
- Gleick's Chaos (excerpt) ওয়েব্যাক মেশিনে আর্কাইভকৃত ২ ফেব্রুয়ারি ২০০৭ তারিখে
- Systems Analysis, Modelling and Prediction Group at the University of Oxford
- A page about the Mackey-Glass equation
- High Anxieties — The Mathematics of Chaos (2008) BBC documentary directed by David Malone
- The chaos theory of evolution – article published in Newscientist featuring similarities of evolution and non-linear systems including fractal nature of life and chaos.
- Jos Leys, Étienne Ghys et Aurélien Alvarez, Chaos, A Mathematical Adventure. Nine films about dynamical systems, the butterfly effect and chaos theory, intended for a wide audience.
- "Chaos Theory", BBC Radio 4 discussion with Susan Greenfield, David Papineau & Neil Johnson (In Our Time, May 16, 2002)
টেমপ্লেট:Systems টেমপ্লেট:Chaos theory টেমপ্লেট:Patterns in nature
